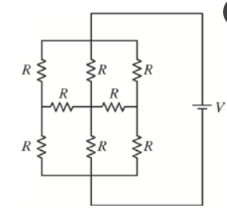
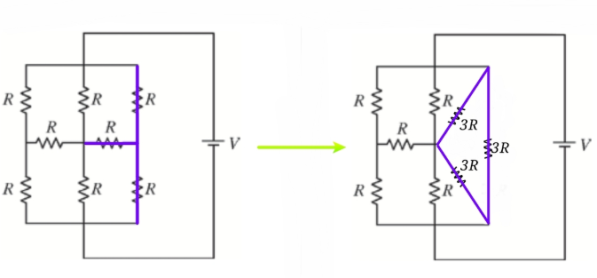
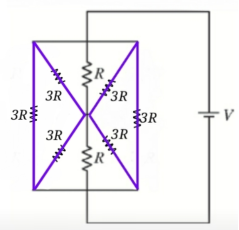
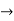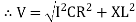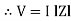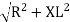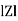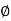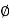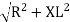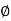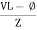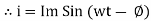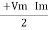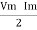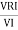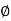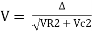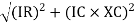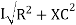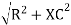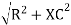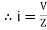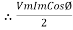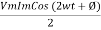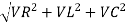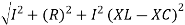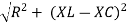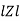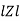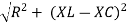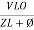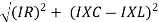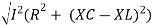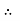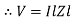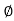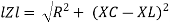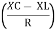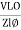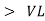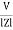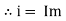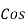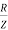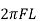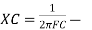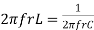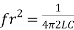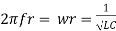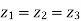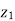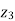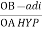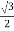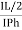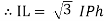|
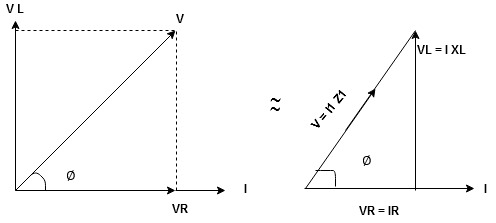
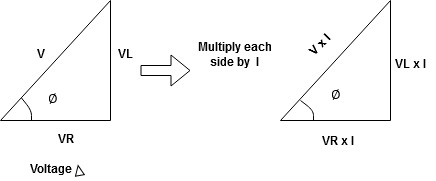
Consider a series R-L circuit connected across voltage source V= Vm sin wt Like some, I is the current flowing through the resistor and inductor due do this current-voltage drops across R and L R
V = IR + I X L
|
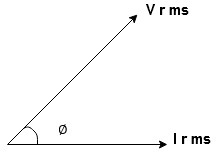
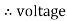 Take current as the reference phasor: 1) for resistor current is in phase with voltage 2) for Inductor voltage leads current or current lags voltage by 90 0.
Take current as the reference phasor: 1) for resistor current is in phase with voltage 2) for Inductor voltage leads current or current lags voltage by 90 0.
|
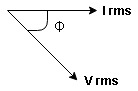
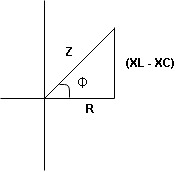
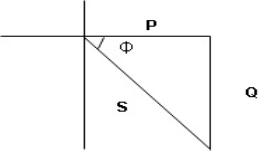
For voltage triangle Ø is the power factor angle between current and resultant voltage V and V =
V =
where Z = Impedance of circuit and its value is |
|
Rectangular form of Z = R+ixL and polar from of Z = (+ j X L + Where
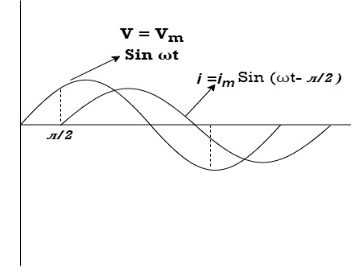
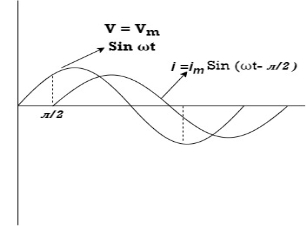
Current Equation : From the voltage triangle, we can sec. that voltage is leading current by Or i =
|
|
|
Power equation P = V .I. P = Vm Sin wt Im Sin wt – Ø P = Vm Im (Sin wt) Sin (wt – Ø) P = Since 2 sin A Sin B = Cos (A-B) – Cos (A+B) P =
①② | |
Average Power pang = Since ② term becomes zero because Integration of cosine come from 0 to 2ƛ
| |
|
From VI = VRI + VLI B Now cos Ø in
Similarly Sin
Apparent Power Average or true Reactive or useless power |
 for R L ekt.
for R L ekt.
|
|
V = Vm sin wt VR
|
|
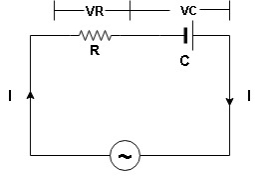
R and C R and C And C
|
|
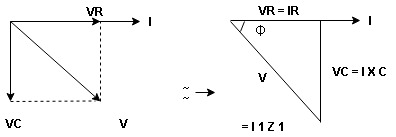
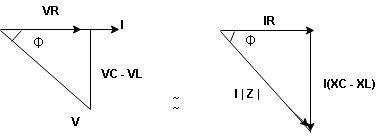
Where Ø is the power factor angle between current and voltage (resultant) V And from voltage V = V = V = V = Where Z = impedance of the circuit and its value is lZl = |
 by
by  as shown
as shown
|
The rectangular form of Z = R - jXc The polar form of Z = lZl L - Ø ( - Ø and –jXc because it is in the fourth quadrant ) where lZl = and Ø = tan -1 | ||
Current equation: from voltage triangle we can see that voltage is lagging current by Ø or current is leading voltage by Ø
Or i =
| ||
|
|
Power Equation: P = V. I P = Vm sin wt. Im Sin (wt + Ø) = Vm Im sin wt sin (wt + Ø) 2 Sin A Sin B = Cos (A-B) – Cos (A+B)
|
Average power
pang = since 2 terms integration of cosine wave from 0 to 2ƛ become zero
|
|
|
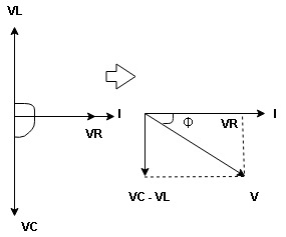
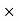 L, VC = I
L, VC = I 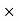 C
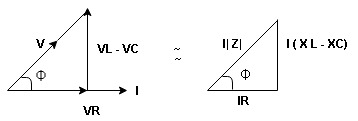
C The voltage drops across XL> than XC
The voltage drops across XL> than XC  VL> VC A
VL> VC A
|
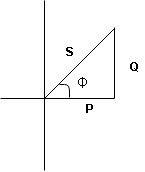
 Resultant voltage triangle
Resultant voltage triangle
|
Now V = VR + VL + VC From voltage triangle V =
|
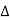 : divide voltage
: divide voltage 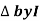
|
Rectangular form Z = R + j (XL – XC) Polor form Z = Where And Ø = tan-1 |
i = i = as VL
|
|
|
 XL :Since we have assured XC
XL :Since we have assured XC  XL
XL  the voltage drops across XC
the voltage drops across XC  than XL
than XL XC
XC  XL (A)
XL (A) voltage triangle considering condition (A)
voltage triangle considering condition (A)
|
 Resultant Voltage
Resultant Voltage 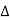
|
Now V = VR + VL + VC From voltage V = V =
|
 Impedance
Impedance 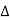 : Divide voltage
: Divide voltage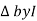
|
Polar form : Z = Where And Ø = tan-1 –
as VC since i =
|
 :
:
|
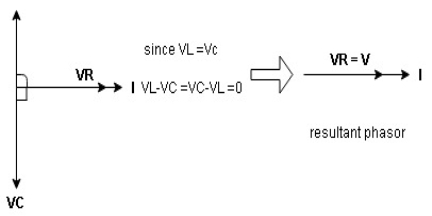
 they will cancel out each other and their resultant will have zero value.Hence resultant V = VR and it will be in phase with I as shown in the below phasor diagram.
they will cancel out each other and their resultant will have zero value.Hence resultant V = VR and it will be in phase with I as shown in the below phasor diagram.
|
From the above resultant phasor diagram V =VR + IR Or V = I Because lZl + R Thus Impedance Z is purely resistive for XL = XC and circuit current will be in phase with source voltage. Since VR=V Øis zero when XL = XC i.e. pang = Vrms I rms cos Ø = 1 cos o = 1 maximum power will be transferred by the condition. XL = XC |
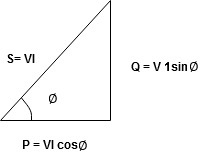
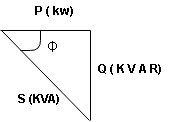
S= V × I Unit - Volte- Ampere (VA) In kilo – KVA
|
P = VI |
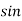 ΦUnit –VA RIn kilo- KVAR
ΦUnit –VA RIn kilo- KVAR
|
i.e. Φ.F= CosΦ |
|
Now Power factor = |
XL =
|
At a particle or frequency f=fr,the inductive and capacitive reactance are exactly Therefore, XL = XC ----at f=fr i.e. Therefore,
|
equal and
|
|
|
i.e. If. |
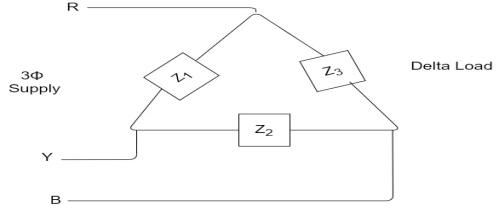
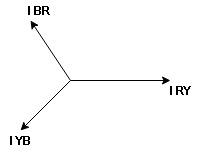
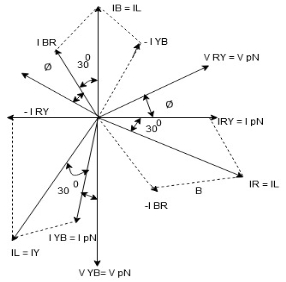
 take phase currents at reference as shown
take phase currents at reference as shown
|
|
Cos 300 =
|
|
|
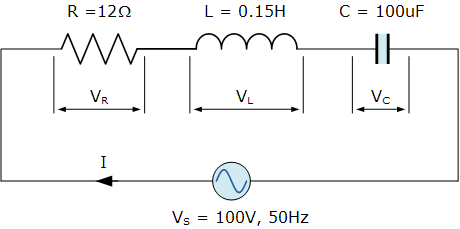
Inductive Reactance, XL. XL = 2 π f L = 2π x 50 x 0.15 = 47.13 Ω
Capacitive Reactance, XC. Xc = 1/ 2π fC = 1/ 2π x 50 x 100 x 10 -6 = 31.83 Ω
Circuit Impedance, Z. Z = [ R 2 + (XL – Xc) 2] ½ Z = [ 12 2 + (47.13 – 31.83) 2] ½ Z = [144 + 234] ½ = 19.4 Ω Circuits Current, I. I = Vs/ Z = 100/ 19.4 = 5.14 amps
Voltages across the Series RLC Circuit, VR, VL, VC. VR = I x R = 5.14 x 12 = 61.7 volts VL = I x XL = 5.14 x 47.13 = 242.2 volts Vc = I x Xc = 5.14 x 31.8 = 163.5 volts |
Circuits Power factor and Phase Angle, θ. cos ɸ = R/Z = 12/ 19.4 = 0.619 cos -1 0.619 = 51.8 lagging Phasor Diagram. | ||
| ||
|
|
|
|