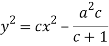Module-4

Ordinary differential equations of first order

Question-1: Solve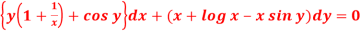

Sol.

Here M = 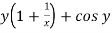and N =

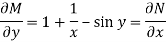
Then the equation is exact and its solution is-

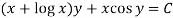
Question-2: Determine whether the differential function ydx –xdy = 0 is exact or not.

Solution.  Here the equation is the form of  M(x , y)dx + N(x , y)dy = 0

  But, we will check for exactness,

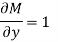
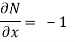
These are not equal results, so we can say that the given diff. Eq. Is not exact.

Question-3: Solve-

Sol.

We can write the given equation as-

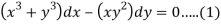
Here,

M =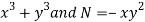

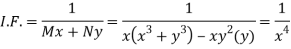
Multiply equation (1) by 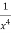we get-

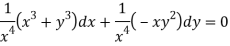
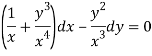
This is an exact differential equation-

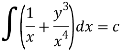
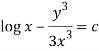
Question-4: Solve-

Sol.

Here given,

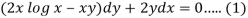
M = 2y and N = 2x log x - xy

Then-

Here,

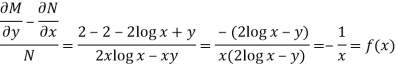
Then,

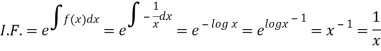
Now multiplying equation (1) by 1/x, we get-

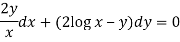
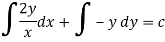
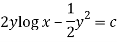
Question-5: Solve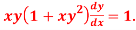

Sol.

We can write the equation as-

On dividing by , we get-

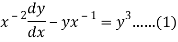
Put 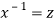so that

Equation (1) becomes,

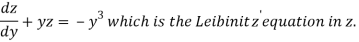
Here,

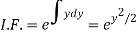
Therefore the solution is-

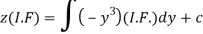
Or

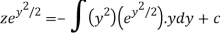
Now put

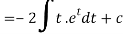
Integrate by parts-

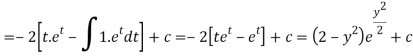
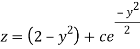
Or
 

Question-6: Solve-

Sol. Here given-

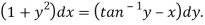
We can re-write this as-

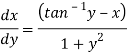
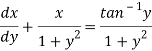
Which is a linear differential equation-

The solution will be-

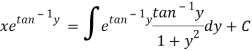
Put

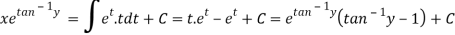
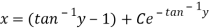
Question-7: find the orthogonal trajectory of the family of curves x² - y² = c

Sol. Here we will follow same procedure as we did in above example,

      Diff. The given equation w.r.t. x, we get

                                              2x – 2y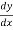  = 0

=

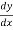Replace 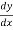  by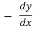

=

= -

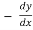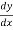Ydy = - xdx

Now integrate the above eq.

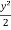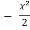   = + c

   On solving we get,

x² + y² = 2c.

Question-8: If a body which is at the temperature of cools down to within 20 minutes. The temperature of the air is .

Find that what will be the temperature of the body after 40 min from its original temperature?

Sol.

Suppose 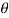 be the temperature of the body at time ‘t’ then-

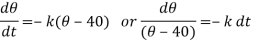
Here k is the constant.

On integrating, we get-

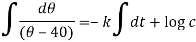
‘c’ is the constant

Or

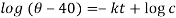
Or

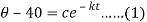
When t = 0, 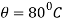and when t = 20 then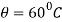

So that- and

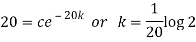
Then equation (1) becomes-

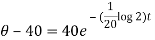
Now, when t = 40 minutes, then-

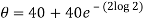
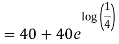
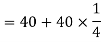
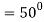
Question-9: Solve-

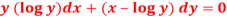
Sol.

We can write the equation as-

We see that it is a Leibnitz’s equation in x-

So that-

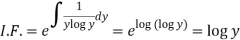
Therefore the solution of equation (1) will be-

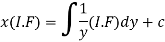
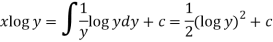
Or

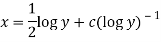
Question-10: Solve-

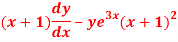
Sol.

Here we have-

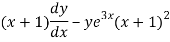
Divide this by (x + 1), we get-

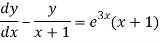
Which is the Leibnitz’s equation-

Here-

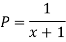
And

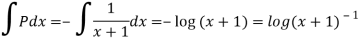
Integrating factor-

The solution will be-

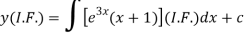
Or

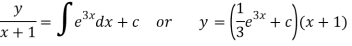
Question-11: Solve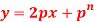

Sol.

Here we have-

Now differentiate it with respect to x, we get-

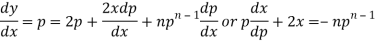
Or

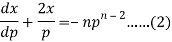
This is the Leibnitz’s linear equation in x and p, here

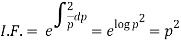
Then the solution of (2) is-

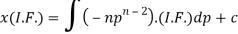
Or

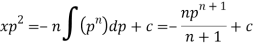
Or

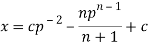
Put this value of x in (1), we get

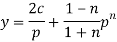
Question-12: Solve-

Sol.

Put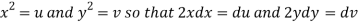

So that-

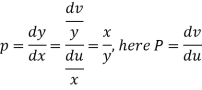
Then the given equation becomes-

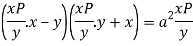
Or

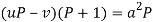
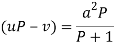
Or

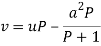
Which is the Clairaut’s form.

Its solution is-

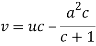
i.e.

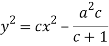
Module-4

Ordinary differential equations of first order

Question-1: Solve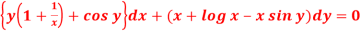

Sol.

Here M = 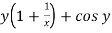and N =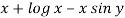

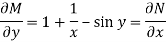
Then the equation is exact and its solution is-

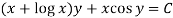
Question-2: Determine whether the differential function ydx –xdy = 0 is exact or not.

Solution.  Here the equation is the form of  M(x , y)dx + N(x , y)dy = 0

  But, we will check for exactness,

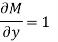
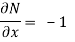
These are not equal results, so we can say that the given diff. Eq. Is not exact.

Question-3: Solve-

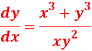
Sol.

We can write the given equation as-

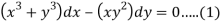
Here,

M =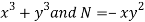

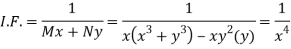
Multiply equation (1) by 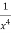we get-

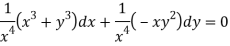
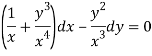
This is an exact differential equation-

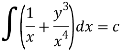
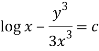
Question-4: Solve-

Sol.

Here given,

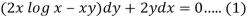
M = 2y and N = 2x log x - xy

Then-

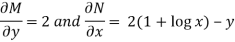
Here,

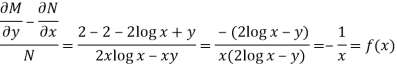
Then,

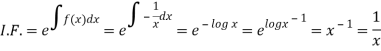
Now multiplying equation (1) by 1/x, we get-

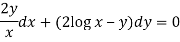
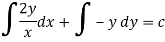
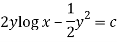
Question-5: Solve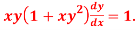

Sol.

We can write the equation as-

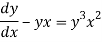
On dividing by 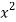, we get-

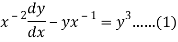
Put 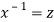so that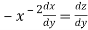

Equation (1) becomes,

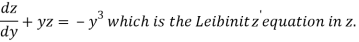
Here,

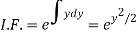
Therefore the solution is-

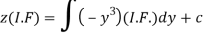
Or

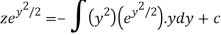
Now put

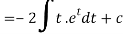
Integrate by parts-

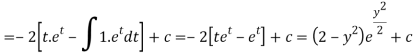
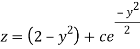
Or
 

Question-6: Solve-

Sol. Here given-

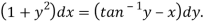
We can re-write this as-

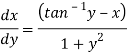
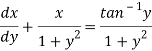
Which is a linear differential equation-

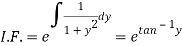
The solution will be-

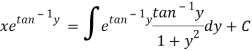
Put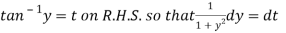

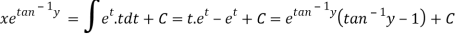
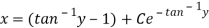
Question-7: find the orthogonal trajectory of the family of curves x² - y² = c

Sol. Here we will follow same procedure as we did in above example,

      Diff. The given equation w.r.t. x, we get

                                              2x – 2y  = 0

=

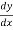Replace   by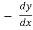

=

= -

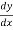Ydy = - xdx

Now integrate the above eq.

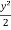   = + c

   On solving we get,

x² + y² = 2c.

Question-8: If a body which is at the temperature of cools down to within 20 minutes. The temperature of the air is .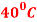

Find that what will be the temperature of the body after 40 min from its original temperature?

Sol.

Suppose 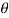 be the temperature of the body at time ‘t’ then-

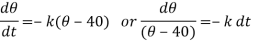
Here k is the constant.

On integrating, we get-

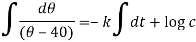
‘c’ is the constant

Or

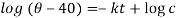
Or

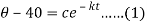
When t = 0, 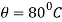and when t = 20 then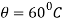

So that- 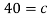and

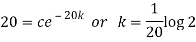
Then equation (1) becomes-

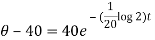
Now, when t = 40 minutes, then-

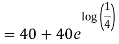
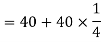
Question-9: Solve-

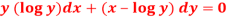
Sol.

We can write the equation as-

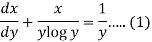
We see that it is a Leibnitz’s equation in x-

So that-

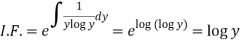
Therefore the solution of equation (1) will be-

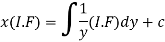
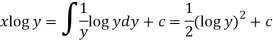
Or

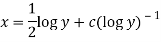
Question-10: Solve-

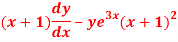
Sol.

Here we have-

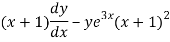
Divide this by (x + 1), we get-

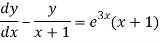
Which is the Leibnitz’s equation-

Here-

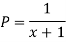
And

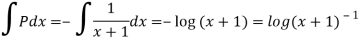
Integrating factor-

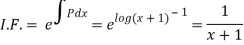
The solution will be-

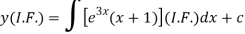
Or

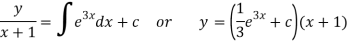
Question-11: Solve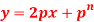

Sol.

Here we have-

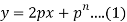
Now differentiate it with respect to x, we get-

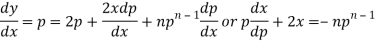
Or

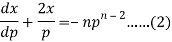
This is the Leibnitz’s linear equation in x and p, here

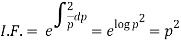
Then the solution of (2) is-

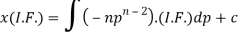
Or

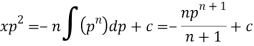
Or

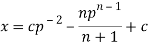
Put this value of x in (1), we get

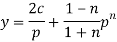
Question-12: Solve-

Sol.

Put

So that-

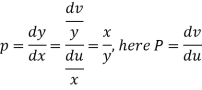
Then the given equation becomes-

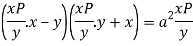
Or

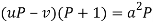
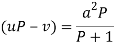
Or

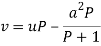
Which is the Clairaut’s form.

Its solution is-

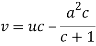
i.e.