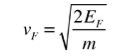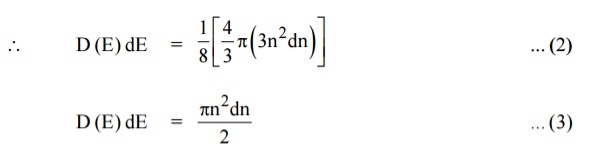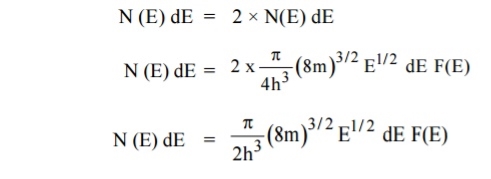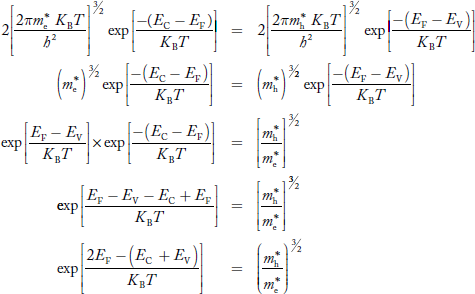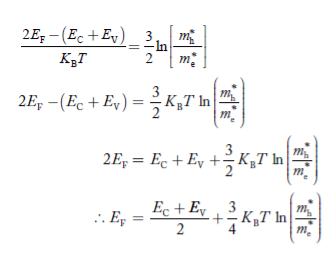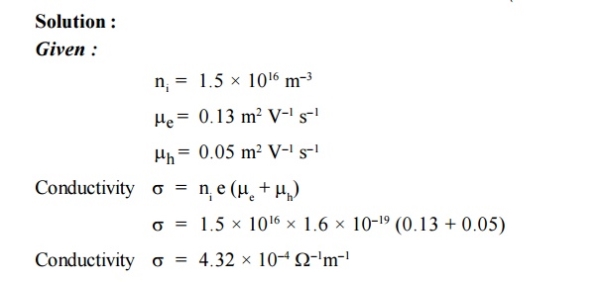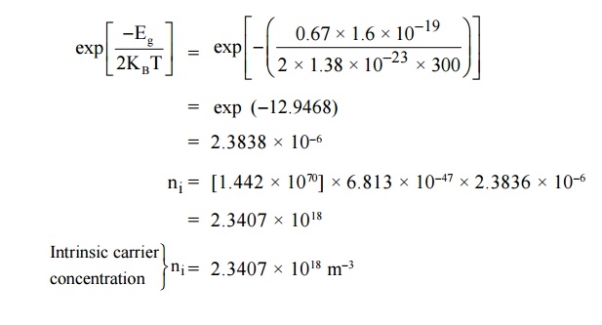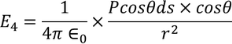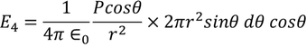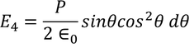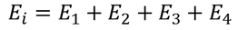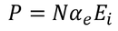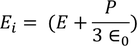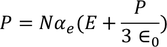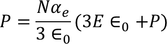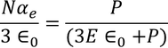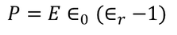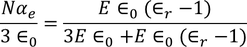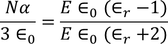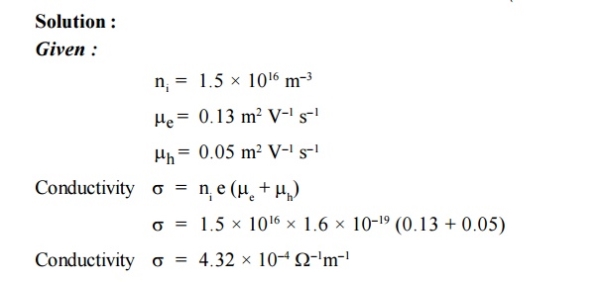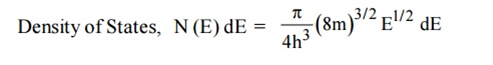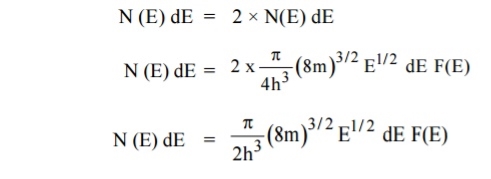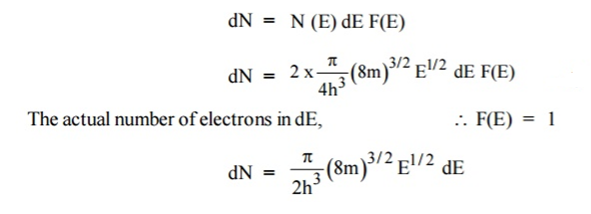Classical Free Electron Theory | Quantum Free Electron Theory |
|
|
2. It is possible that many electron may possess same energy.
| 2. The free-electrons obey the Pauli exclusion principle. Hence no two electrons can possess same energy. |
3. The pattern of distribution of energy among the free electron obey Maxwell-Boltzmann statistics.
| 3. The distribution of energy among the free electrons is according to Fermi Dirac statistics which imposes a severe restriction on the possible way in which the electrons absorb energy from an external source. |
 kT. It is related to the kinetic energy equation
kT. It is related to the kinetic energy equation  kT =
kT = 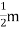 v2thHere vth represents the thermal velocity. Q3) Write Success and failure of classical free electron theory?A3) Success of classical free electron theory1. It verifies ohm’s law 2. It explains electrical conductivity of metals. 3. It explains thermal conductivity of metals. 4. It derives Widemann – Franz law. (i.e. the relation between electrical and thermal conductivity. Draw backs of classical free electron theory. 1. It could not explain the photoelectric effect, Compton Effect and black body radiation. 2. Electrical conductivity of semiconductors and insulators could not be explained. 3. Widemann – Franz law (K/σT= constant) is not applicable at lower temperatures. 4. Ferromagnetism could not be explained by this theory. The theoretical value of paramagnetic susceptibility is greater than the experimental value. 5. According to classical free electron theory the specific heat of metals is given by 4.5R whereas the experimental value is given by 3R. 6. According to classical free electron the electronic specific heat is equal to 3/2R while the actual value is 0.01R.Q4) Derive expression for Electrical Conductivity according to free electron theory and also discuss its temperature dependence?A4) The classical free electron theory was proposed by Drude and Lorentz. According to this theory the electrons are moving freely and randomly moving in the entire volume of the metal like gas atoms in the gas container. When an electric field is applied the free electrons gets accelerated. When an electric field E is applied between the two ends of a metal of area of cross section A When an electrical field (E) is applied to an electron of charge ‘e’ of a metallic rod, the electron moves in opposite direction to the applied field with a velocity vd. This velocity is known as drift velocity. Drift velocity vd is defined as the average velocity of the free electrons with which they move towards the positive terminal under the influence of the electrical field. Lorentz force acting on the electron F = eE ........(1) This force is known as the driving force of the electron.Due to this force, the electron gains acceleration ‘a’. From Newton’s second law of motion, Force F = ma …....(2) From the equation (1) and (2),ma = eE or a =
v2thHere vth represents the thermal velocity. Q3) Write Success and failure of classical free electron theory?A3) Success of classical free electron theory1. It verifies ohm’s law 2. It explains electrical conductivity of metals. 3. It explains thermal conductivity of metals. 4. It derives Widemann – Franz law. (i.e. the relation between electrical and thermal conductivity. Draw backs of classical free electron theory. 1. It could not explain the photoelectric effect, Compton Effect and black body radiation. 2. Electrical conductivity of semiconductors and insulators could not be explained. 3. Widemann – Franz law (K/σT= constant) is not applicable at lower temperatures. 4. Ferromagnetism could not be explained by this theory. The theoretical value of paramagnetic susceptibility is greater than the experimental value. 5. According to classical free electron theory the specific heat of metals is given by 4.5R whereas the experimental value is given by 3R. 6. According to classical free electron the electronic specific heat is equal to 3/2R while the actual value is 0.01R.Q4) Derive expression for Electrical Conductivity according to free electron theory and also discuss its temperature dependence?A4) The classical free electron theory was proposed by Drude and Lorentz. According to this theory the electrons are moving freely and randomly moving in the entire volume of the metal like gas atoms in the gas container. When an electric field is applied the free electrons gets accelerated. When an electric field E is applied between the two ends of a metal of area of cross section A When an electrical field (E) is applied to an electron of charge ‘e’ of a metallic rod, the electron moves in opposite direction to the applied field with a velocity vd. This velocity is known as drift velocity. Drift velocity vd is defined as the average velocity of the free electrons with which they move towards the positive terminal under the influence of the electrical field. Lorentz force acting on the electron F = eE ........(1) This force is known as the driving force of the electron.Due to this force, the electron gains acceleration ‘a’. From Newton’s second law of motion, Force F = ma …....(2) From the equation (1) and (2),ma = eE or a = 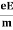 …......(3)Acceleration (a) =
…......(3)Acceleration (a) = 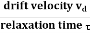 Or a =
Or a =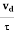 Relaxation time is defined as the time taken by a free electron to reach its equilibrium position from the disturbed position in the presence of an electric field.So vd = a ……….(4)Substituting equation (3) in (4)vd =
Relaxation time is defined as the time taken by a free electron to reach its equilibrium position from the disturbed position in the presence of an electric field.So vd = a ……….(4)Substituting equation (3) in (4)vd = 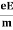 =
= 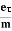 E ……….(5)vd =
E ……….(5)vd = 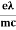 E ……….(5a)Where
E ……….(5a)Where  = RMS velocityThe Ohms’ law states that current density (J) is expressed as J =E ……….(6)Or =
= RMS velocityThe Ohms’ law states that current density (J) is expressed as J =E ……….(6)Or =  Where is the electrical conductivity of the electron. But, the current density in terms of drift velocity is given as J = nevd ....... (7)Substituting equation (5) in equation (7), we haveJ = ne
Where is the electrical conductivity of the electron. But, the current density in terms of drift velocity is given as J = nevd ....... (7)Substituting equation (5) in equation (7), we haveJ = ne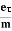 E Or
E Or  =
= 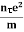 On comparing the equation (6) and (8) , we haveElectrical conductivity =
On comparing the equation (6) and (8) , we haveElectrical conductivity =  ....... (8) =
....... (8) = 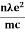 ....... (8a)This is a required expression for electrical conductivity.Resistivity ρρ =
....... (8a)This is a required expression for electrical conductivity.Resistivity ρρ = 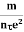 =
= 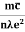 According to kinetic theory of gasses
According to kinetic theory of gasses =
= 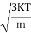 So =
So =  =
=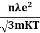 Alsoρ =
Alsoρ = 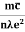 =
= 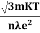 Mobility: It is defined as the drift velocity of the charge carrier per unit applied electric field.μ=
Mobility: It is defined as the drift velocity of the charge carrier per unit applied electric field.μ= 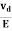 ....... (9)From equation (7) we have J = nevdby substituting vd =μEfrom equation (9)J = neμEOr
....... (9)From equation (7) we have J = nevdby substituting vd =μEfrom equation (9)J = neμEOr  = μne ....... (10)We know by ohms law =
= μne ....... (10)We know by ohms law =  so equation (10) can be rewritten as= μneμ =
so equation (10) can be rewritten as= μneμ =  This is the required expression for mobility.Temperature dependenceThe positive ions are always in oscillating (or vibrating) state about their mean position; even the substance is present at 0k temperature. The vibrating amplitude of ions is always depends the temperature. The mean free path λ of the electrons is inversely proportional to the mean square of amplitude of ionic vibrations A0λ =
This is the required expression for mobility.Temperature dependenceThe positive ions are always in oscillating (or vibrating) state about their mean position; even the substance is present at 0k temperature. The vibrating amplitude of ions is always depends the temperature. The mean free path λ of the electrons is inversely proportional to the mean square of amplitude of ionic vibrations A0λ = 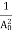 ………(1) The energy of lattice vibrations is proportional to
………(1) The energy of lattice vibrations is proportional to 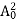 and increases linearly with temperature T.
and increases linearly with temperature T. 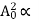 T ……….(2) From equations (1) and (2)λ =
T ……….(2) From equations (1) and (2)λ =  ………..(3) The resistivity ρ of the metal is inversely proportional to mean free path of electrons λρ =
………..(3) The resistivity ρ of the metal is inversely proportional to mean free path of electrons λρ =  ..…………..(4) From equations (3) and (4)ρ
..…………..(4) From equations (3) and (4)ρ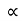 T ..……….(5) From equation (5) we observe that the resistivity of metal is linearly increases with temperature. The conductivity is defined as the reciprocal of resistance.
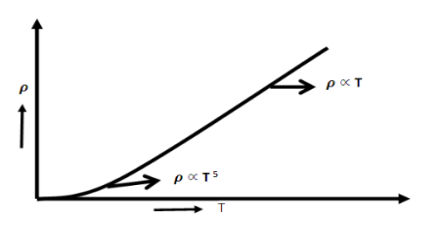
T ..……….(5) From equation (5) we observe that the resistivity of metal is linearly increases with temperature. The conductivity is defined as the reciprocal of resistance. 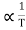 ………….(6) From equation (6) we observe that the conductivity of metal is inversely proportional to their temperature. The variation of resistivity of metal with temperature is shown in figure.
………….(6) From equation (6) we observe that the conductivity of metal is inversely proportional to their temperature. The variation of resistivity of metal with temperature is shown in figure.
|
|
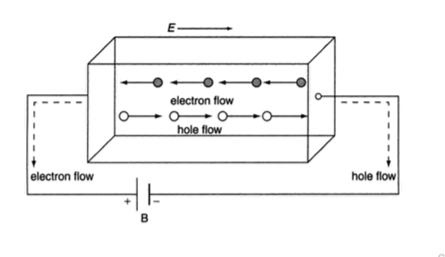
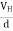 ……………..(4)In n-type material, Jx = –nevdvd = -
……………..(4)In n-type material, Jx = –nevdvd = - 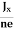 ……………..(5)Where n is free electron concentration, substituting (5) in (3), we have∴ EH = -B
……………..(5)Where n is free electron concentration, substituting (5) in (3), we have∴ EH = -B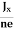 ……………..(6)For a given semiconductor, the Hall field EH is proportional to the current density Jx and the intensity of magnetic field ‘B’ in the material.i.e. EH ∝ JxB(or) EH = RHJxB ……………..(7)Where RH = Hall coefficientEquations (6) and (7) are same so, we haveRHJxB =-B
……………..(6)For a given semiconductor, the Hall field EH is proportional to the current density Jx and the intensity of magnetic field ‘B’ in the material.i.e. EH ∝ JxB(or) EH = RHJxB ……………..(7)Where RH = Hall coefficientEquations (6) and (7) are same so, we haveRHJxB =-B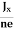 RH = -
RH = -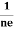 = -
= - ……………..(8)Where ρ is charge density Similarly for p-type materialRH =
……………..(8)Where ρ is charge density Similarly for p-type materialRH =  =
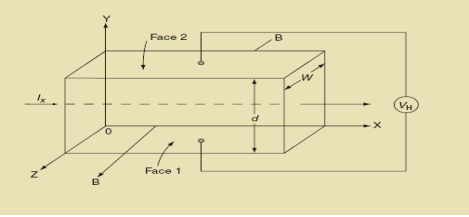
= ……………..(9)Using Equations (8) and (9), carrier concentration can be determined.Thus, the Hall coefficient is negative for n-type material. In n-type material, as more negative charge is deposited at the bottom surface, so the top face acquires positive polarity and the Hall field is along negative Y-direction. The polarity at the top and bottom faces can be measured by applying probes. Similarly, in case of p-type material, more positive charge is deposited at the bottom surface. So, the top face acquires negative polarity and the Hall field is along positive Y-direction. Thus, the sign of Hall coefficient decides the nature of (n-type or p-type) material. The Hall coefficient can be determined experimentally in the following way:Multiplying Equation (7) with ‘d’, we haveEHd = VH = RHJxBd ……………..(10)From (Figure 11) we know the current density JxJx =
……………..(9)Using Equations (8) and (9), carrier concentration can be determined.Thus, the Hall coefficient is negative for n-type material. In n-type material, as more negative charge is deposited at the bottom surface, so the top face acquires positive polarity and the Hall field is along negative Y-direction. The polarity at the top and bottom faces can be measured by applying probes. Similarly, in case of p-type material, more positive charge is deposited at the bottom surface. So, the top face acquires negative polarity and the Hall field is along positive Y-direction. Thus, the sign of Hall coefficient decides the nature of (n-type or p-type) material. The Hall coefficient can be determined experimentally in the following way:Multiplying Equation (7) with ‘d’, we haveEHd = VH = RHJxBd ……………..(10)From (Figure 11) we know the current density JxJx = 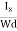 Where W is width of the box. Then, Equation (10) becomesVH = RH
Where W is width of the box. Then, Equation (10) becomesVH = RH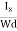 Bd = RH
Bd = RH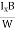 RH =
RH =  ……………..(11)Substituting the measured values of VH, Ix, B and W in Equation(11), RH is obtained. The polarity of VH will be opposite for n- and p-type semiconductors.The mobility of charge carriers can be found by using the Hall effect, for example, the conductivity of electrons isn= neμnOr we can rewrite it asμn =
……………..(11)Substituting the measured values of VH, Ix, B and W in Equation(11), RH is obtained. The polarity of VH will be opposite for n- and p-type semiconductors.The mobility of charge carriers can be found by using the Hall effect, for example, the conductivity of electrons isn= neμnOr we can rewrite it asμn = 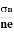 =n RH ……………..(12)by using equation (11)μn = n
=n RH ……………..(12)by using equation (11)μn = n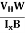 ……………..(13) Q6) Explain the term Fermi level and Fermi energy?A6) Fermi level" is the term used to describe the top of the collection of electron energy levels at absolute zero temperature. This concept comes from Fermi-Dirac statistics. Electrons are fermions and by the Pauli Exclusion Principle cannot exist in identical energy states. So at absolute zero they pack into the lowest available energy states and build up a "Fermi sea" of electron energy states. The Fermi level is the surface of that sea at absolute zero where no electrons will have enough energy to rise above the surface. The concept of the Fermi energy is a crucially important concept for the understanding of the electrical and thermal properties of solids. Both ordinary electrical and thermal processes involve energies of a small fraction of an electron volt. But the Fermi energies of metals are on the order of electron volts. This implies that the vast majority of the electrons cannot receive energy from those processes because there are no available energy states for them to go to within a fraction of an electron volt of their present energy. Limited to a tiny depth of energy, these interactions are limited to "ripples on the Fermi sea". At higher temperatures a certain fraction, characterized by the Fermi function, will exist above the Fermi level. The Fermi level plays an important role in the band theory of solids. In doped semiconductors, p-type and n-type, the Fermi level is shifted by the impurities, illustrated by their band gaps. The Fermi level is referred to as the electron chemical potential in other contexts.
……………..(13) Q6) Explain the term Fermi level and Fermi energy?A6) Fermi level" is the term used to describe the top of the collection of electron energy levels at absolute zero temperature. This concept comes from Fermi-Dirac statistics. Electrons are fermions and by the Pauli Exclusion Principle cannot exist in identical energy states. So at absolute zero they pack into the lowest available energy states and build up a "Fermi sea" of electron energy states. The Fermi level is the surface of that sea at absolute zero where no electrons will have enough energy to rise above the surface. The concept of the Fermi energy is a crucially important concept for the understanding of the electrical and thermal properties of solids. Both ordinary electrical and thermal processes involve energies of a small fraction of an electron volt. But the Fermi energies of metals are on the order of electron volts. This implies that the vast majority of the electrons cannot receive energy from those processes because there are no available energy states for them to go to within a fraction of an electron volt of their present energy. Limited to a tiny depth of energy, these interactions are limited to "ripples on the Fermi sea". At higher temperatures a certain fraction, characterized by the Fermi function, will exist above the Fermi level. The Fermi level plays an important role in the band theory of solids. In doped semiconductors, p-type and n-type, the Fermi level is shifted by the impurities, illustrated by their band gaps. The Fermi level is referred to as the electron chemical potential in other contexts.In metals, the Fermi energy gives us information about the velocities of the electrons which participate in ordinary electrical conduction. The amount of energy which can be given to an electron in such conduction processes is on the order of micro-electron volts (see copper wire example), so only those electrons very close to the Fermi energy can participate. The Fermi velocity of these conduction electrons can be calculated from the Fermi energy.
This speed is a part of the microscopic Ohm's Law for electrical conduction. For a metal, the density of conduction electrons can be implied from the Fermi energy. |
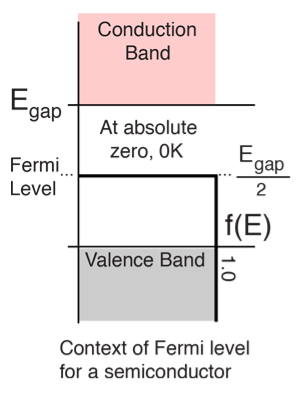
Figure 4: Fermi level for a Semiconductor
|
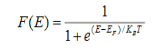 Where KB Boltzmann Constant T Temperature If g(E) is the density of states and f(E) gives the probability of occupation of those states at a given temperature, the number of occupied states, n(E),is given by n(E) =
Where KB Boltzmann Constant T Temperature If g(E) is the density of states and f(E) gives the probability of occupation of those states at a given temperature, the number of occupied states, n(E),is given by n(E) = 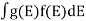 (1) If the number of occupied states in an energy band needs to be calculated the integration needs to be performed over the entire band. This will be a function of temperature, since the Fermi function is temperature dependent. Equation 1 can be used to calculate the concentration of electron and holes in semiconductors, which decides their conductivity.Effect of temperature on Fermi Function:Case (i) Probability of occupation for E < EF at T = 0K
(1) If the number of occupied states in an energy band needs to be calculated the integration needs to be performed over the entire band. This will be a function of temperature, since the Fermi function is temperature dependent. Equation 1 can be used to calculate the concentration of electron and holes in semiconductors, which decides their conductivity.Effect of temperature on Fermi Function:Case (i) Probability of occupation for E < EF at T = 0K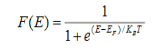 When T = 0K and E < EF, we haveF(E) =
When T = 0K and E < EF, we haveF(E) = 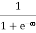 =
= 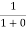 = 1Thus at T = 0K, there is 100 % chance for the electrons to occupy the energy levels below the Fermi level.
= 1Thus at T = 0K, there is 100 % chance for the electrons to occupy the energy levels below the Fermi level.
|
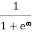 =
= 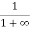 =
=  = 0Thus, there is 0 % chance for the electrons to occupy energy levels above the Fermi energy level. From the above two cases, at T = 0K the variation of F(E) for different energy values becomes a step function.Case (iii) Probability of occupation at ordinary temperatureAt ordinary temperature, the value of probability starts reducing from 1 for values of E slightly less than EF. With the increase of temperature, i.e., T> 0K, Fermi function F (E) varies with E. At any temperature other than 0K and E = EFF(E) =
= 0Thus, there is 0 % chance for the electrons to occupy energy levels above the Fermi energy level. From the above two cases, at T = 0K the variation of F(E) for different energy values becomes a step function.Case (iii) Probability of occupation at ordinary temperatureAt ordinary temperature, the value of probability starts reducing from 1 for values of E slightly less than EF. With the increase of temperature, i.e., T> 0K, Fermi function F (E) varies with E. At any temperature other than 0K and E = EFF(E) = 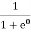 =
= 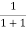 =
=  = 50%Hence, there is 50 % chance for the electrons to occupy Fermi level. Further, for E > EF the probability value falls off rapidly to zero. Case (iv) At high temperatureWhen kT >> EF, the electrons lose their quantum mechanical character and Fermi distribution function reduces to classical Boltzmann distributionQ8) Derive relation for density of states?A8) A parameter of interest in the study of conductivity of metals and semiconductors is the density of states. The Fermi function F(E) gives only the probability of filling up of electrons in a given energy state. It does not give the information about the number of electrons that can be filled in a given energy state, to know that we should know the number of available energy states called density of states.Density of states is defined the as the number of energy states per unit volume in an energy interval of a metal. It is use to calculate the number of charge carriers per unit volume of any solid.
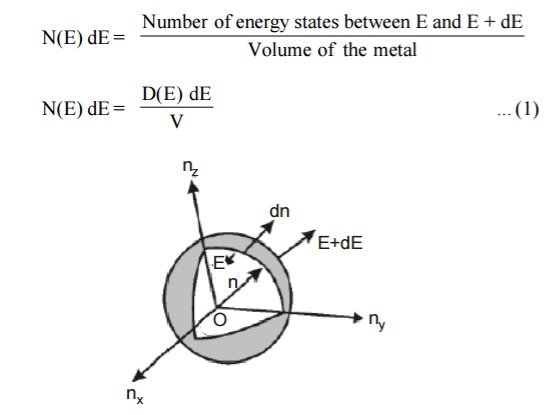
= 50%Hence, there is 50 % chance for the electrons to occupy Fermi level. Further, for E > EF the probability value falls off rapidly to zero. Case (iv) At high temperatureWhen kT >> EF, the electrons lose their quantum mechanical character and Fermi distribution function reduces to classical Boltzmann distributionQ8) Derive relation for density of states?A8) A parameter of interest in the study of conductivity of metals and semiconductors is the density of states. The Fermi function F(E) gives only the probability of filling up of electrons in a given energy state. It does not give the information about the number of electrons that can be filled in a given energy state, to know that we should know the number of available energy states called density of states.Density of states is defined the as the number of energy states per unit volume in an energy interval of a metal. It is use to calculate the number of charge carriers per unit volume of any solid.
|
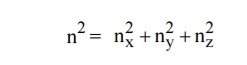 The sphere is further divided into many shells represents a particular combination of quantum numbers and represents particular energy value.Therefore, the number of energy states within a sphere of radius
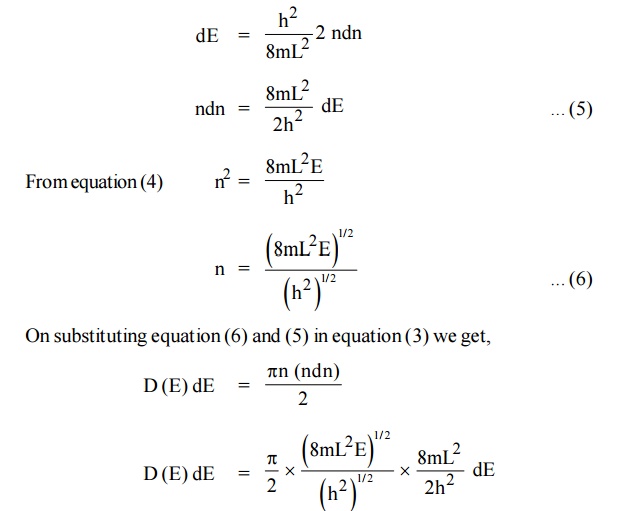
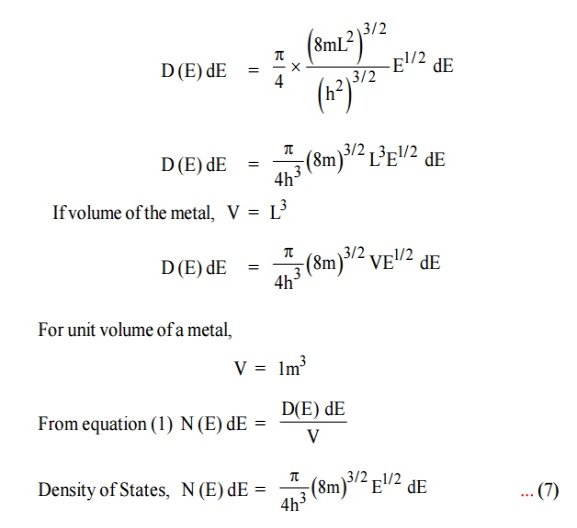
The sphere is further divided into many shells represents a particular combination of quantum numbers and represents particular energy value.Therefore, the number of energy states within a sphere of radius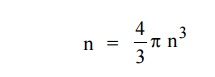 Let us consider two energy values E and E + dE can be found by finding the number of energy states between the shells of radius n and n+ dn from the origin. Since the quantum numbers are positive integers, n values can be defined only in the positive octant of the n – space. The number of available energy states within the sphere of radius “n” due to one octant.
Let us consider two energy values E and E + dE can be found by finding the number of energy states between the shells of radius n and n+ dn from the origin. Since the quantum numbers are positive integers, n values can be defined only in the positive octant of the n – space. The number of available energy states within the sphere of radius “n” due to one octant.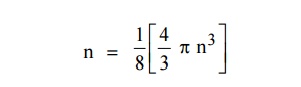 Similarly the number of available energy states within the sphere of radius n+dn corresponding energy.
Similarly the number of available energy states within the sphere of radius n+dn corresponding energy.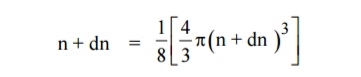 The number of available energy states between the shells of radius n and n + dn (or) between the energy levels E and E + dE
The number of available energy states between the shells of radius n and n + dn (or) between the energy levels E and E + dE
|
|
|
|
|
|
|
|
|
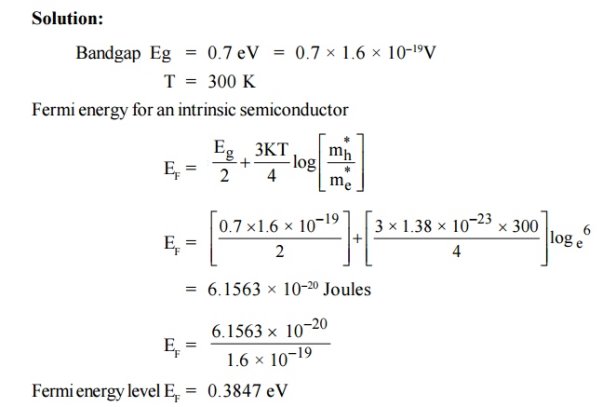
 is very small so that EF is just lie in the middle of energy gap Temperature effect on Fermi levelFermi level slightly rises with increase of temperature.But in case of pure intrinsic semiconductor like Si and Ge, mh* ≈ me* so in these cases Fermi level lies at the middle of energy gap.Q10) The intrinsic carrier density is 1.5 × 1016 m–3. If the mobility of electron and hole are 0.13 and 0.05 m2 V–1 s–1, calculate the conductivity.A10.
is very small so that EF is just lie in the middle of energy gap Temperature effect on Fermi levelFermi level slightly rises with increase of temperature.But in case of pure intrinsic semiconductor like Si and Ge, mh* ≈ me* so in these cases Fermi level lies at the middle of energy gap.Q10) The intrinsic carrier density is 1.5 × 1016 m–3. If the mobility of electron and hole are 0.13 and 0.05 m2 V–1 s–1, calculate the conductivity.A10.
|
|
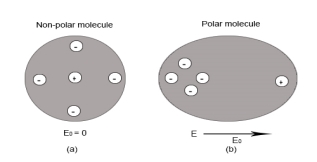
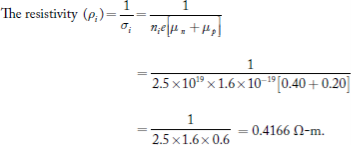 Q12) What are the differences between polar and non-polar molecules? Also discuss the effect of electric field on dielectric? A12) Polar Molecules: Polar Molecules are those in which centre of gravity of positive and negative charge does not coincide with each other. This is because they all are asymmetric in shape. Examples: H2O, CO2, NO2 etc.Non-Polar Molecule: Non-polar molecules are those in which centre of gravity of positive charge and negative charge coincide with each other. The molecule has zero dipole moment as they all are symmetric in shape. Examples: O2, N2, H2 etc.
Q12) What are the differences between polar and non-polar molecules? Also discuss the effect of electric field on dielectric? A12) Polar Molecules: Polar Molecules are those in which centre of gravity of positive and negative charge does not coincide with each other. This is because they all are asymmetric in shape. Examples: H2O, CO2, NO2 etc.Non-Polar Molecule: Non-polar molecules are those in which centre of gravity of positive charge and negative charge coincide with each other. The molecule has zero dipole moment as they all are symmetric in shape. Examples: O2, N2, H2 etc.
|
|
|
a) Magnetic Field
b) Electric field
c) Vacuum
d) Inert EnvironmentA13) b
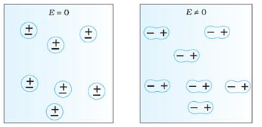
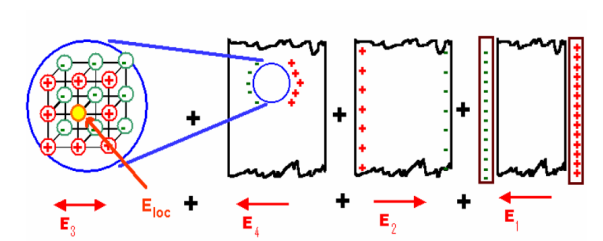
When the dielectric is placed in an electric field, like between the parallel plates of a capacitor, the dipoles are created and they tend to align themselves parallel to the direction of the electric field.Q14) Derive Claussius -Mosotti equation?A14) In dielectric solids, the atoms or molecules experience not only the external applied electric field but also the electric field produced by the dipoles. The resultant electric field acting on the atoms or molecules of dielectric substance is called the local field or an internal field.To find an expression for local electric field on a dielectric molecule or an atom, we consider a dielectric material in the electric field of intensity E, between the capacitor plates so that the material is uniformly polarized, as a result opposite type of charges are induced on the surface of the dielectric near the capacitor plates. The local field is calculated by using the method suggested by Lorentz.According to this method, Internal field or Local field in solidsConsider a dielectric material and is subjected to external field of intensity E1. The charges are induced on the dielectric plate and the induced electric field intensity is taken as E2. Let E3 be the field at the center of the material. E4 be the induced field due to the charges on the spherical cavity. The total internal field of the material is
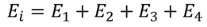 ………..(1)
………..(1)
|
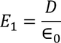 ………..(2)We know
………..(2)We know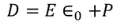 ………..(3)Substituting the Electric flux density D in E1, we get
………..(3)Substituting the Electric flux density D in E1, we get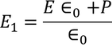 ………..(4)
………..(4) 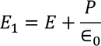 ………..(5)E2 is the Electric field intensity due to induced or polarized charges
………..(5)E2 is the Electric field intensity due to induced or polarized charges 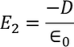 ………..(6)Here the charge is induced due to the induced field so the electric flux density D changes to the electric polarization P
………..(6)Here the charge is induced due to the induced field so the electric flux density D changes to the electric polarization P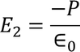 ………..(7)Since we have considered that the specimen is non-polar dielectric material, at the center of the specimen the dipole moment is zero and hence the electric field intensity at the center is zero due to symmetric structure.
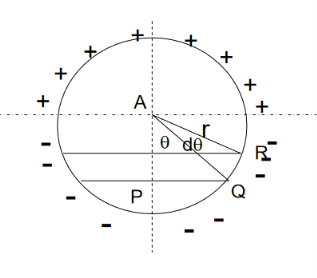
………..(7)Since we have considered that the specimen is non-polar dielectric material, at the center of the specimen the dipole moment is zero and hence the electric field intensity at the center is zero due to symmetric structure.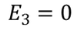 ………..(8)Now consider a circle from the center of the dielectric material. Calculation of the electric field intensity E4 on the surface of spherical cavity.As we know the polarization P is the induced charge per unit area
………..(8)Now consider a circle from the center of the dielectric material. Calculation of the electric field intensity E4 on the surface of spherical cavity.As we know the polarization P is the induced charge per unit area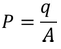 ………..(9) Here the polarization changes to its component so we will take the component which is contributing.
………..(9) Here the polarization changes to its component so we will take the component which is contributing. 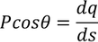
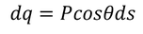 ………..(10)Now this equation can be solved by finding out the values of the charge dq in the surface are dA. We know the Electric field intensity E
………..(10)Now this equation can be solved by finding out the values of the charge dq in the surface are dA. We know the Electric field intensity E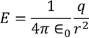 ………..(11)Multiplying with the cosine angle on both the sides we get
………..(11)Multiplying with the cosine angle on both the sides we get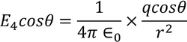 ………..(12)Now by applying all the present condition for the above equation we
………..(12)Now by applying all the present condition for the above equation we 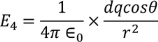 ………..(13)Now substituting the charge dq in the above equation we get
………..(13)Now substituting the charge dq in the above equation we get
|
|
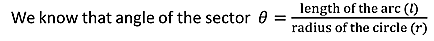 Hence dA= 2πrsinθrdθ= 2πr2sinθdθ ………..(17)Now substituting all the values in the electric field intensity on the spherical cavity E4 we get,
Hence dA= 2πrsinθrdθ= 2πr2sinθdθ ………..(17)Now substituting all the values in the electric field intensity on the spherical cavity E4 we get,
Substituting (17) in (18), we get
Integrating with in the limits 0 to π
On solving the integration we get,
So the total electric field
Hence the Internal field obtained is
|
we know internal field
From above equations
we know polarization from the relation between polarization and dielectric constant
from the above two equations we get,
|
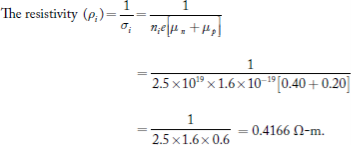 Q16) The intrinsic carrier density is 1.5 × 1016 m–3. If the mobility of electron and hole are 0.13 and 0.05 m2 V–1 s–1, calculate the conductivity.A16)
Q16) The intrinsic carrier density is 1.5 × 1016 m–3. If the mobility of electron and hole are 0.13 and 0.05 m2 V–1 s–1, calculate the conductivity.A16)
|
|
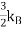 where
where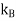 , is the Boltzmann constant. If we have N number of atomsand each give one valence electron to the electron gas, and the electrons are freely mobile, then the electronic contribution to the heat capacity should be
, is the Boltzmann constant. If we have N number of atomsand each give one valence electron to the electron gas, and the electrons are freely mobile, then the electronic contribution to the heat capacity should be 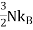 , same as for the case of atoms of a monatomic gas. But the observed electronic contribution at room temperature is usually less than this value. How can the electrons participate in electrical conduction processes as if they were mobile, while not contributing to the heat capacity?This important discrepancy is removed with the help of Quantum theory of free electrons. It was discovered that the specific heat vanishes at absolute zero and that at low temperatures it is proportional to the absolute temperature.When we heat the specimen from absolute zero, not every electron gains energy
, same as for the case of atoms of a monatomic gas. But the observed electronic contribution at room temperature is usually less than this value. How can the electrons participate in electrical conduction processes as if they were mobile, while not contributing to the heat capacity?This important discrepancy is removed with the help of Quantum theory of free electrons. It was discovered that the specific heat vanishes at absolute zero and that at low temperatures it is proportional to the absolute temperature.When we heat the specimen from absolute zero, not every electron gains energy 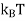 as expected classically, but only those electrons in orbitals within an energy range
as expected classically, but only those electrons in orbitals within an energy range 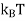 of the Fermi level are excited thermally. This gives an immediate qualitative solution to the problem of the heat capacity of the conduction electron gas. If N is the total number of electrons, only a fraction of the order of T/TF can he excited thermally at temperature T,This is because only these electrons lie within an energy range of the order of
of the Fermi level are excited thermally. This gives an immediate qualitative solution to the problem of the heat capacity of the conduction electron gas. If N is the total number of electrons, only a fraction of the order of T/TF can he excited thermally at temperature T,This is because only these electrons lie within an energy range of the order of 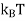 of the top of the energy distribution.Each of these NT/TF electrons has a thermal energy of the order of kBT.The total electronic thermal kinetic energy U is of the order ofU
of the top of the energy distribution.Each of these NT/TF electrons has a thermal energy of the order of kBT.The total electronic thermal kinetic energy U is of the order ofU 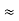 (NT/TF)
(NT/TF) 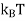 The electronic heat capacity is given byC =
The electronic heat capacity is given byC = 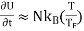 and is directly proportional to T, in agreement with the experimental results discussed in the following section. At room temperature C is smaller than the classical value
and is directly proportional to T, in agreement with the experimental results discussed in the following section. At room temperature C is smaller than the classical value 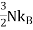 , by a factor of the order of 0.01 or less, for TF
, by a factor of the order of 0.01 or less, for TF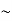 5
5 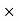 104K.2. This theory explains photo electric effect, Compton Effect and block body radiation etc.3. This theory gives the correct mathematical expression for the thermal conductivity of metalsThe temperature-dependent part of the electrical resistivity is proportional to the rate at which an electron collides with thermal phonons and thermal electrons. The collision rate with phonons is proportional to the concentration of thermal phonons. Here the phonon concentration is proportional to the temperature T. So using quantum theory result the thermal conductivity can be calculated.Failures of Quantum free electron theory1. This theory fails to explain bands presence and also not able to distinguish between metal, semiconductor and Insulator2. According to this theory, only two electrons are present in the Fermi level and they are responsible for conduction which is not true.3. Quantum free electron theory not able to explain the positive value of Hall Co-efficient. Q20) Give assumptions of quantum free electron theory?A20) The failure of classical free electron theory paved this way for Quantum free electron theory. It is introduced by Sommerfeld in 1928. Arnold Sommerfeldovercomes many drawbacks of the classical free electron theory by applying quantum mechanical principles in 1928.Quantization of electrical energy levels is added here. He also used the Pauli Exclusion Principle in restricting the energy values of electron. His theory is known as Quantum Free Electron TheoryThe main assumptions of quantum free electron theory are,
104K.2. This theory explains photo electric effect, Compton Effect and block body radiation etc.3. This theory gives the correct mathematical expression for the thermal conductivity of metalsThe temperature-dependent part of the electrical resistivity is proportional to the rate at which an electron collides with thermal phonons and thermal electrons. The collision rate with phonons is proportional to the concentration of thermal phonons. Here the phonon concentration is proportional to the temperature T. So using quantum theory result the thermal conductivity can be calculated.Failures of Quantum free electron theory1. This theory fails to explain bands presence and also not able to distinguish between metal, semiconductor and Insulator2. According to this theory, only two electrons are present in the Fermi level and they are responsible for conduction which is not true.3. Quantum free electron theory not able to explain the positive value of Hall Co-efficient. Q20) Give assumptions of quantum free electron theory?A20) The failure of classical free electron theory paved this way for Quantum free electron theory. It is introduced by Sommerfeld in 1928. Arnold Sommerfeldovercomes many drawbacks of the classical free electron theory by applying quantum mechanical principles in 1928.Quantization of electrical energy levels is added here. He also used the Pauli Exclusion Principle in restricting the energy values of electron. His theory is known as Quantum Free Electron TheoryThe main assumptions of quantum free electron theory are,
|
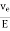 orμeE =
orμeE = 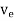 substituting in equation 2Je =neμeE ..........(3)From Ohms law, J = E where Je = eEJe =neμeE = eE ..........(4)e = neμe ..........(5)Similarly current density for holesJp =peμpE = pE ..........(6)And conductivityp = peμp ..........(7)Substituting value of Je and Jp from eq 4 and 6 in eq 1, we getJ = neμeE + peμpE J = (neμe + peμp)E ..........(8)From Ohms law J = E = neμe + peμpwheree = neμe andp = peμpAlso ni =CT3/2e -Eg/2kT ..........(9)Therefore = nie(μe + μp) = CT3/2e -Eg/2kT e(μe + μp) ..........(10)The mobilities of carrier depend upon temperature asμ
substituting in equation 2Je =neμeE ..........(3)From Ohms law, J = E where Je = eEJe =neμeE = eE ..........(4)e = neμe ..........(5)Similarly current density for holesJp =peμpE = pE ..........(6)And conductivityp = peμp ..........(7)Substituting value of Je and Jp from eq 4 and 6 in eq 1, we getJ = neμeE + peμpE J = (neμe + peμp)E ..........(8)From Ohms law J = E = neμe + peμpwheree = neμe andp = peμpAlso ni =CT3/2e -Eg/2kT ..........(9)Therefore = nie(μe + μp) = CT3/2e -Eg/2kT e(μe + μp) ..........(10)The mobilities of carrier depend upon temperature asμ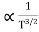 For Electrons μe
For Electrons μe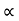
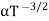 and for holes μp
and for holes μp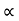
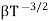 μe + μp=
μe + μp=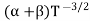 =
= 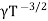 ..........(11)Using equation 11 in equation 10 = CT3/2e -Eg/2kT e
..........(11)Using equation 11 in equation 10 = CT3/2e -Eg/2kT e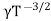 = C e
= C e e -Eg/2kTLet B = C e
e -Eg/2kTLet B = C e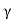 we get = Be -Eg/2kT ..........(12) Q22) Derive relation for Fermi energyand show Fermi energy of a metal depends only on the density of electrons of that metal?A22) Let us recall the formula for density of states (we derived density of states under article 5.4, equation 7)
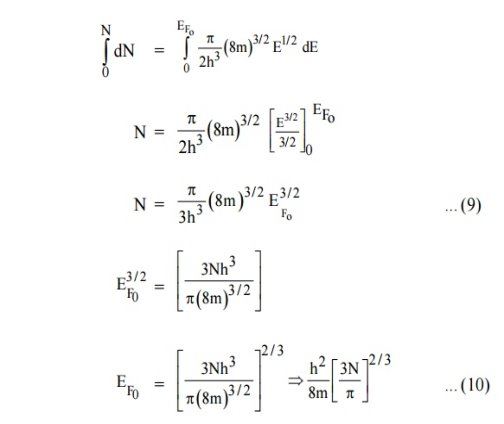
we get = Be -Eg/2kT ..........(12) Q22) Derive relation for Fermi energyand show Fermi energy of a metal depends only on the density of electrons of that metal?A22) Let us recall the formula for density of states (we derived density of states under article 5.4, equation 7)
|
|
|
|