B.Tech 5th Sem Exam
Heat Transfer
Q.1 Choose the correct answer of the following:
a. Which of the following is the case of heat transfer by radiation?
- Blast furnace
- Heating of building
- Cooling of parts in furnace
- Heat received form a person by fireplace
- All of above
Answer: Heat received form a person by fireplace
b. On a heat transfer surface, fins are provided to
- Increase temperature gradient so as to enhance heat transfer
- Increase turbulence in flow for enhancing heat transfer
- Increase surface area to promote the rate of heat transfer
- Decrease the pressure drop of the fluid
Answer: Increase surface area to promote the rate of heat transfer
c. Consider two walls, A, B with the same surface area and the same temp. Drop across their thickness. The ratio of K is KA/KB = 4. And the ratio of LA/LB = 02. The ratio heat transfer rate through the walls QA/QB is
- 0.5
- 1
- 2
- 4
Answer: 0.5
d. The thermal resistance of a hollow cylinder is given as
- Ln (Ro / Ri) / 2πkl
- Ln (Ri / Ro) / 2πkl
- (Ro + Ri) / 2πkl
- (RoRi) / 2πkl
Answer: ln (Ro / Ri) / 2πkl
e. The Biot number can be thought of as the ratio of
- The conduction thermal resistance to the convective thermal resistance.
- The convective thermal resistance to the conduction thermal resistance.
- The thermal energy storage capacity to the conduction thermal resistance.
- The thermal energy storage capacity to the convection thermal resistance.
Answer: The conduction thermal resistance to the convective thermal resistance.
f. The free convection heat transfer is significantly affected by
- Reynolds number
- Grashoff number
- Prantdtl number
- Stanton number.
Answer: Grashoff number
g. In a counter flow heat exchanger cold fluid enters at 30°C and leaves at 50°C whereas the hot fluid enters at 150°C and leaves at 130°C. The mean temperature difference for this case.
- 20
- 60
- 100
- Indeterminate
Answer: Indeterminate
h. What is the basic equation of thermal radiation from which all other equations of radiation can be derived?
- Stefan-Boltzmann equation
- Planck’s equation
- Wien’s equation
- Rayleigh-Jeans formula
Answer: Planck’s equation
i. The hydrodynamic and thermal boundary layer are identical at prandtl no equal to
- 0.5
- 1
- 2
- 5
Answer: 1
j. The automobile radiator is a heat exchanger of.
- Parallel flow type
- Counter flow type
- Cross flow type
- Regenerator type
Answer: Cross flow type
Q.2 a. What are the different modes of heat transfer? How does heat conduction differ from heat convection?
Answer:
There are three modes of heat transfer.
- Conduction
- Convection
- Radiation
1. Conduction of Heat
Heat conduction is a process in which heat is transferred from the hotter part to the colder part in a body without involving any actual movement of the molecules of the body. Heat transfer takes place from one molecule to another molecule as a result of the vibratory motion of the molecules. Heat transfer through the process of conduction occurs in substances which are in direct contact with each other. It generally takes place in solids.
Conduction example: When frying vegetables in a pan. Heat transfer takes place from flame to the pan and then to the vegetables.
Based on the conductivity of heat, substances can be classified as conductors and insulators. Substances that conduct heat easily are known as conductors and those that do not conduct heat are known as insulators.
2. Convection of Heat
In this process, heat is transferred in the liquid and gases from a region of higher temperature to a region of lower temperature. Convection heat transfer occurs partly due to the actual movement of molecules or due to the mass transfer.
For example. Heating of milk in a pan.
3. Radiation of Heat
It is the process in which heat is transferred from one body to another body without involving the molecules of the medium. Radiation heat transfer does not depend on the medium.
For example: In a microwave, the substances are heated directly without any heating medium.
Complete step by step answer:
Conduction | Convection |
The mechanism of heat transfer from the hot body to the cold body due to free electrons is heat transfer by conduction. | The mechanism in which heat transfer in fluids is due to the physical movement of molecules is convection heat transfer. |
Owing to the difference in temperature, the heat transfer takes place. | The flow of heat happens regardless of the disparity in mass. |
In conduction, the heat transfer is sluggish. | The heat transfer in the convection phase is quicker. |
b. State the Fourier’s law of heat conduction.
Answer:
It is known that the conduction of heat takes place when the molecules of matter vibrate or agitate and transmit energy to the adjacent molecules. As the neighbouring molecules collide, heat energy is transferred from a higher temperature area to a lower one. This process abides by Fourier’s law. So let us go through the article below to understand what is Fourier’s law. Fourier’s law is also called the law of thermal conduction equations or the law of thermal conductivity. This law is taught in schools in the NCERT book that follow the CBSE curriculum. In order to understand this law index, there are certain concepts that students should learn and not understand like Newton's law of cooling, ohm's law, heat transfer, change of state, specific heat capacity, measurement of temperature.
Fourier’s law is also known as the law of heat conduction, it mainly states that the heat transfer rate through a material is considered to be proportional to the negative gradient present in the temperature, as well as to the area which is at right angles to the gradient, through which the heat flows. This law has two equivalent forms- integral form and differential form. This law is considered to be in an empirical relationship that is based on observation.
c. Generation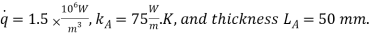 
The wall material B has no generation with kB = 150 W/m . K and thickness LB = 20 mm. The inner surface of material A is well insulated, while the outer surface of material B is cooled by a water stream with T = 30o C and h = 1000 W/m2. K
Determine the temperature of the insulated surface and the temperature of the cooled surface.
Answer:
Given : A composite wall of material A and B :
gA = 2.5 106 W/m3
kA = 110 W/m. K
LA = 60 mm = 0.06 m
gB = 0
kB = 150 W/m.K
LB = 20 mm = 0.02 m
T = 30o C
h = 1000 W/m2.K
To find :
(i) Temperature distribution in the composite.
(ii) Temperature of insulated surface of A and cooled surface of material B.
Assumptions:
(i) Steady state heat conduction in axial direction only,
(ii) Negligible contact resistance at interface.
(iii) Constant properties.
Analysis: (i) (a) the temperature distribution in material A is given as
TA(x) = -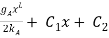 
It is parabolic temperature distribution in material A as shown in
Fig. And it is subjected to boundary conditions
At x = 0, the slope dT/dx = 0
And at x = LA, TA(x) = T2
(b) The temperature distribution in material B is given as
TB(x) = C3x + C4
It is a linear distribution between temperatures T2 and T3.
(c) Large gradient near wall B due to water cooling.
(ii) The heat flux in wall material A can be calculated as
q = gA LA = 2.5 106 0.06
= 150 103 W/m2
Since inner surface of material A is well insulated and hence under steady state, this heat must be dissipated from outer surface of material B to water stream
Thus q = h(T3 - T)
Or T3 – q/h + T
= ( 150 103)/1000 + 30 = 180o C
It is the temperature of cooled surface of material B.
The temperature T2 at interface of two material can be calculated as
q = kB(T2- T3)/LB
Or T2 = qLB/kB + T3
( 150 103 0.02)/150 + 180 = 200oC
Now temperature distribution in material A
TA (x) = gAx2/2kA + C1x + C2
Subjected boundary conditions:
At x = 0, dT/dx = 0
It gives C1 = 0
And at x = LA, T = T2
Or T2 = - gALA2/2kA + C2
It gives C2 = T2 = gALA2/2kA
Then TA(x) = gA/2kA (LA2 – x2) + T2
= 
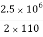(0.062 – x2) + 200
= 11363.63 (0.062 – x2) + 200
The inner surface temperature of material A, (x = 0)
T1 = 11363.63 (0.06)2 + 200
= 240.9oC
Q.3 a. Adding insulation on a cylindrical surface will always decrease heat transfer rate.
True or False Explain.
Answer: True
In a plane wall, the area perpendicular to the direction of heat flow adding more insulation to a wall always decreases heat transfer. The thicker the insulation, the lower the heat transfer rate, and this is because the outer surface always has the same area.
But in cylindrical and spherical coordinates, the addition of insulation also increases the outer surface, which decreases the convection resistance at the outer surface. Moreover, in some cases, a decrease in the convection resistance due to the increase in surface area can be more significant than an increase in conduction resistance due to thicker insulation. As a result, the total resistance may actually decrease, resulting in increased heat flow.
The thickness up to which heat flow increases and after which heat flow decreases is termed critical thickness. In the case of cylinders and spheres, it is called the critical radius. The critical radius of insulation can be derived depending on the thermal conductivity of the insulation k and the external convection heat transfer coefficient h.
b. In cylindrical fuel element for gas-cooled nuclear reactor; the generation rate of thermal energy within the fuel element due to fission can be approximated by the relation q(r) qol 1 - (2) Wlm? where a is the radius of the fuel element and % is constant The boundary surface at r = a is maintained at a uniform temperature To: (a) Assuming one-dimensional, steady-state heat flow; develop relation for the temperature drop from the centerline t0 the surface of the fuel element (b) For a radius of a = 30 mm, the thermal conductivity k = 10 WI(m *C) and % 2 X 107 Wlm?, calculate the temperature drop from the centerline t0 the surface.
Answer:
Given:
(i) A cylindrical fuel element with heat generation
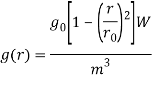(ii) Outer surface at uniform temperature T0.
Mathematical Formulation:
Recognition of Problem:
(i) Heat is generated in the fuel element.
(ii) No time dependent quantity is given.
(iii) The outer surface of fuel element is maintained at uniform temperature To.
(iv) Heat conduction in one dimension. These conditions indicate for one dimensional steady state heat conduction with heat generation.
Its governing equation in radial direction is given by eqn.
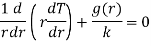
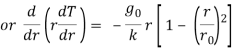
Subjected to boundary conditions
(i) At r = r0, T = T0
(ii) For solid rod in steady state, the temperature
Gradient at centre is always zero due to symmetry
i.e., at r = 0, dT/dr = 0
Where T = f(r)
Q.4 a. What is boundary conditions? Mention the different types of boundary conditions.
Answer:
Differential equations have many solutions and it’s usually impossible to find them all. To narrow down the set of answers from a family of functions to a particular solution, conditions are set. These conditions can be initial conditions (which define a starting point at the extreme of an interval) or boundary conditions (which define bounds that constrain the whole solution). Different types of boundary conditions can be imposed on the boundary of a domain.
One way to think of the difference between the two is that initial conditions deal with time, while boundary conditions deal with space. Boundaries can describe all manner of shapes: e.g. Triangles, circles, polygons.
Types of Boundary Conditions
The five types of boundary conditions are:
- Dirichlet (also called Type I),
- Neumann (also called Type II, Flux, or Natural),
- Robin (also called Type III),
- Mixed,
- Cauchy.
Dirichlet and Neumann are the most common.
- Dirichlet: Specifies the function’s value on the boundary. For example, you could specify Dirichlet boundary conditions for the interval domain [a, b], giving the unknown at the endpoints a and b. For two dimensions, the boundary conditions stretch along an entire curve; for three dimensions, they must cover a surface. This type of problem is called a Dirichlet Boundary Value Problem..
- Neumann: Similar to the Dirichlet, except the boundary condition specifies the derivative of the unknown function. For example, we could specify u′(a) = α which imposes a Neumann boundary condition at the right endpoint of the interval domain [a, b].
- Robin: A weighted combination of the function’s value and its derivative. For example, for unknown u(x) on the interval domain [a, b] we could specify the Robin condition u(a) −2u′(a) = 0.
- Mixed: Similar to the Robin, except that parts of the boundary are specified by different conditions. For example, on the interval [a, b], the unknown u′(x) at x = a could be specified by a Neumann condition and the unknown u(x) at x = b could be specified by a Dirichlet condition.
- Cauchy: Similar to the Robin, except that while the Robin condition implies only one constraint, the Cauchy condition implies two.
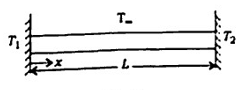
(b) A thin fin of length L has its two ends fixed to two parallel walls at temperatures T1 and T2, the temperature of the environment being T. Show that the expression for one-dimensional temperature distribution along the length of the fin can be represented in the Fig. 1 below :
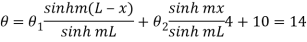
Q.5 a. What are the inherent dimensionless parameters for forced convection?
Answer:
Forced convection is a mechanism, or type of transport, in which fluid motion is generated by an external source (like a pump, fan, suction device, etc.). Alongside natural convection, thermal radiation, and thermal conduction it is one of the methods of heat transfer and allows significant amounts of heat energy to be transported very efficiently.
The following dimensionless parameters plays a significant role for evaluating the convection heat transfer coefficient (h):
- It is a dimensionless quantity defined as: hL/ k,
Where h = convective heat transfer coefficient
L is the characteristic length
k is the thermal conductivity of the fluid.
The following dimensionless parameters plays a significant role for evaluating the convection heat transfer coefficient (h):
- It is a dimensionless quantity defined as: hL/ k,
Where h = convective heat transfer coefficient
L is the characteristic length
k is the thermal conductivity of the fluid.
- The Nusselt number is the ratio of the temperature gradient in the fluid for its immediate contact with the surface to a reference temperature gradient (Ts - T∞) /L.
- The convective heat transfer coefficient (h) can be find if the Nusselt number (Nu), the thermal conductivity of the fluid (kf) in that temperature range and the characteristic dimension of the object (Lc) are given.
- Let the hot flat plate having temperature Tw is placed in a free stream such that T∞ < Tw. The temperature distribution is shown below in the figure.
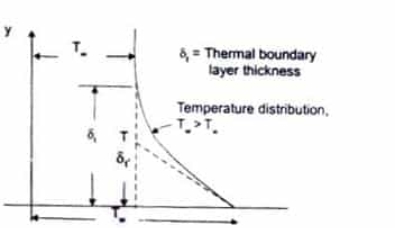
b. In a fully developed region of flow in a circular tube will the velocity profile change in the flow direction? How about the temperature profile
Answer:
The thickness of the boundary layer inside a pipe increases in the flow direction until it reaches the pipe center and it fills the entire pipe. A little further downstream the velocity becomes fully developed and remains constant. It occurs when the normalized temperature profile remains unchanged as the pipe is not heated or cooled during the flow process.
Therefore in the fully developed region of flow in a circular pipe, the velocity profile does not change in the flow direction.
(c) Consider the velocity and temperature profiles for a fluid dlow in a tube with diameter of 50 mm can be expressed as
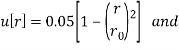
T(r) = 400 + 80 (r/R)2 – 30(r/R)3
With units in m/s and K, respectively. Determine the average velocity and the mean temperature from the given velocity and temperature profiles.
Answer:
Q 6. a. What is the physical significance of biot number? Represents diagrammatically the effect of Ni on steady state temperature distribution in a plane wall with surface convection.
- Biot number and Fourier number are two dimensionless number used in transient heat transfer.
- They are mainly used in transient heat transfer, where you want to find the time of cooling/heating of the object from a temperature to ambient temperature.
- Example- 100°C (iron) metal ball is cooling in constant temperature air at 30°C. If someone wants to know the temperature at a time or want to find the time to reach the temperature to a particular value, the transient heat with Lumped system is considered provided the practical value Biot number is less than 0.01 (Theoretically it should be zero)
- Biot number is the ratio of conductive heat transfer resistance of the object material to convective heat transfer resistance of the fluid
- Bi=hLckBi=hLck
- Hh - heat transfer coefficient of the medium
- LcLc - Characteristics length of the object in that configuration
- Kk - Conductivity of the object material.
- Fourier number is defined as
- Fo = αt/Lc2
- α – Thermal diffusivity of the material
- α = k/cp
- - density of the body
- k – Conductivity of the body
- For Lumped system analysis the equation is given by
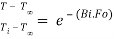
- Bi. Fo = hLc/k kt/cpLc2
- T - Ambient temperature
- Ti – initial temperature of the body
- T – Temperature of the body at any instant t seconds after the cooling/heating process is started.
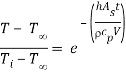
- This equation can be simplified to
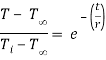
- Where Τ = cpLc/h called time constant.
- Here V/As is known as Lc
- V – Volume of the body
- As – Total Surface area of the body
- For sphere Lc = D/6
- For cube Lc = a/6
- For Long Cylinder Lc = D/4
b. A long cylinder of radius 150 mm and at an initial uniform temperature of 530 ° C is suddenly exposed to an environment at 30⁰C . The convection heat transfer coefficient between the surface of the cylinder and the environment is 380W/(m^2K ) . The thermal conductivity and thermal diffusivity of the cylinder material are 200W/(mK) and 8.5×10^-5m^2/s respectively . Determine ( a ) the temperature at a radius of 120 mm and ( b ) the heat transferred per metre length of the cylinder 265 seconds after the cylinder is exposed to the environment ( use Heisler charts ) .
Answer:
The temperature distribution in a solid cylinder of radius R with uniform internal heat generation is given by
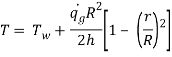
Where,
Tw = temperature at the outer radius (R), 
k = thermal conductivity
T = Temperature at radius r
At steady state, heat going out of the cylinder by conduction is absorbed by the atmosphere through convection whose temperature is T (Tw > T)
Heat conducted:
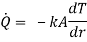
At r = R
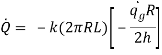
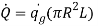
Heat convected
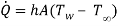
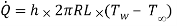
At steady – state heat conducted is equal to heat convected:
qg(πR2L) = h 2πRL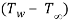 
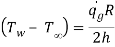
Given heat flux is q0;
q0 =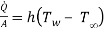 
q0 = 
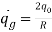
Q. 7 a. What do you mean by fully developed flow? Explain with suitable sketch.
Answer:
Let's consider the flow of fluid in the pipe from tank
As the fluid enters the pipe by virtue of no slip condition boundary layer will happen and the fluid will acquire the velocity of pipe adjacent to pipe
As we move further in the radial direction the velocity in the center of the pipe will increase and the velocity profile will tend to acquire the shape of parabola from the flat profile at the entrance point.
After we get the parabola shape of velocity profile…even if we move further along the length of pipe velocity profile remains same that is du/dx=0 after that cross section
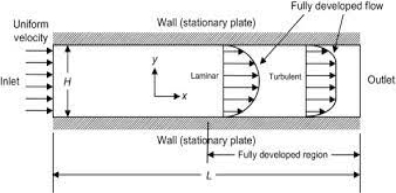
From the entrance point up to the perfect parabolic shape it is known as entrance length or entrance region. It is the distance travelled by the flow before it becomes fully developed. Beyond this region we will get a fully developed flow.
Also at the starting cross section of fully developed flow two boundary layers of pipe meet
For laminar flow to know the length of entrance region use the formula L/d=0.06*Reynolds no
For turbulent flow L/d=4.4*Reynolds no to the power 1/6
Where L=entrance length d=dia of pipe
b. Derive the 2-D differential form of conservation of energy equation for the boundary layer of laminar, incompressible flow over a flat plate with constant fluid properties.
Answer:
The time-averaged differential equation for energy in a given flow field is linear in the temperature if fluid properties are considered to be independent of temperature. Thus, the concept of a Heat Transfer Coefficient arises such that the heat transfer rate from a wall is given by:
(1)
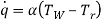
Where the heat transfer coefficient, α, is only a function of the flow field. Tw is the wall temperature and Tr, the recovery or adiabatic wall temperature. The above is also true of the Boundary Layer energy equation, which is a particular case of the general energy equation. When fluids encounter solid boundaries, the fluid in contact with the wall is at rest and viscous effects thus retard a layer in the vicinity of the wall. For large Reynolds Numbers based on distance from the leading edge, these viscous layers are thin compared to this length.
When the wall is at a different temperature to the fluid, there is similarly a small region where the temperature varies. These regions are the velocity and thermal boundary layers. In 1905 Prandtl showed that this thin region could be analyzed separately from the bulk fluid flow in that pressure variation normal to the wall may be neglected and the pressure is given by that impressed by the free stream. Velocity normal to the wall is also of order, of the thickness of the boundary layer, the characteristic velocity being that of the free stream and the length being the distance from the leading edge. Thus, the boundary layer equations for steady incompressible laminar flow in two dimensions may be approximated to be:
(2)
(3)
(4)
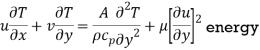
p, T, u and v are the flow pressure, temperature and velocities along and perpendicular to the surface, respectively. λ, μ, cp and ρ are similarly the thermal conductivity, viscosity, specific heat and density. x and y are Cartesian coordinates along and perpendicular to the surface.
The classical laminar solution to the momentum equation was provided by Blasius for the case of a semi-infinite flat plate aligned with uniform flow. The velocities normalized by the free-stream value u0 are plotted in Figure 1 vs. The nondimensional quantity η = y/xRex−1/2 η = y/x Rex-1/2 ; Rex is the Reynolds number based on distance from the leading edge of the plate.
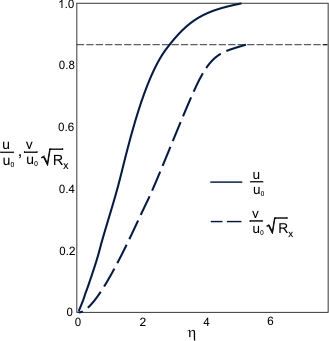
Figure 1. Laminar boundary layer normalized velocities along and perpendicular to a flat plate from Young (1989).
The velocity gradient at the wall gives the skin friction, τ, which can be expressed as the skin friction coefficient:
(5)
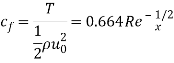
The suffix o refers to the free-stream value.
The energy equation may be solved using the Blasius solution to give the heat transfer in terms of the Nusselt Number, Nux, when the dissipation term, μ(∂u/∂y)2 is neglected.
(6)
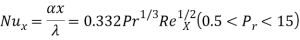
This may also be expressed in terms of a Stanton Number, St = α/ρucp, as, Re St Pr = Nu. Figure 2 shows temperature profiles for different Prandtl Numbers. There is thus an analogy between heat transfer skin friction (Reynolds analogy) which may be expressed as:
(7)
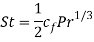
Expressions for the boundary layer deficit thicknesses of mass, momentum and temperature are, respectively:
(8)
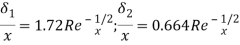
(9)
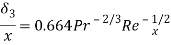
The deficit thickness represents the height of free-stream fluid which carries the boundary layer deficit in the relevant quantity
(10)
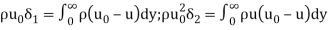
(11)
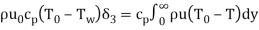
c. Physically, what does the Grashof number represents?
Answer:
Grashof number is a dimensionless quantity which is significant in case of fluid flow due to natural convection. Free/natural convection is the mode of heat transfer arising due to density difference in the fluid. Grashof number is the ratio of the buoyancy force to viscous force.
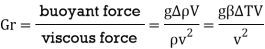
Higher the value of Grashof number, higher is the buoyancy force which means the fluid movement is high.
While Reynolds number is the ratio of inertial force to viscous force. Reynolds number is used in forced convection problems where the flow is induced by an external source such as pump, fan, suction device etc…
In dimensional analysis,
For forced convection, Nu = f(Re, Pr)
For natural/free convection, Nu = f(Gr, Pr)
Q.8 a. What is fouling factor? Explain its effect in heat exchanger design.
Answer:
Heat exchanger fouling factor quantifies the effect of fouling in the reduced heat transfer efficiency. It is also often referred to as the 'dirt factor'. It normally depends on the process fluid or service on any side (shell or tube) of the heat exchanger.
Fouling or dirt factor is used for calculation of the overall heat transfer coefficient of a heat exchanger. Consider the following equation.
Where, U = Overall heat transfer coefficient
Ho = Shell side heat transfer coefficient
Hi = Tube side heat transfer coefficient
Rdo = shell side fouling factor
Rdi = tube side fouling factor
OD and ID are respectively the outer and internal diameters for the selected tube size
Ao and Ai are outer and inner surface area values for the tubes
kw is the resistance value for the tube wall
It is clear from the equation that, as the fouling factors (either on shell or tube side) increase, overall heat transfer coefficient will decrease. In other words, heat exchanger fouling reduces the overall heat transfer rate.
b. Define heat exchanger effectiveness.
Answer:
The heat exchange process between two fluids with different temperatures using solid walls occurs in various engineering applications. The tool to achieve this exchange is a heat exchanger. Some applications like air conditioning, power generation, waste heat recovery, and chemical processing use this device.
The basis of the work of a heat exchanger is that the hot fluid enters the heat exchanger at temperature T1 and its heat capacity is Chot. Also, the cold fluid with the heat capacity of Ccold enters temperature t1; in the meantime, the hot fluid loses its heat, and its temperature drops to T2. It delivers heat to the cold fluid to increase its temperature to t2 and leave the heat exchanger at this temperature.
A simple schematic of a heat exchanger
In general, the efficiency of heat exchange is defined as the ratio of the amount of heat transferred in the actual case to the amount of heat in the ideal case, in the optimal case (equation below).
The heat transfer rate in the actual state is defined as the basis of the rate of change of cold or hot fluid temperature.
(c) Derive for parallel flow heat exchanger
ε =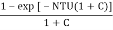 
Where, C = Cmin/Cmax, NTU = Number of transfer units.
4 + 2+8 = 14
Assuming the outer surface of the heat exchanger to be well insulated so that any heat transfer occurs between the two fluids, and disregarding any changes in kinetic and potential energy, an energy balance on each fluid in a differential section of the heat exchanger can be expressed as
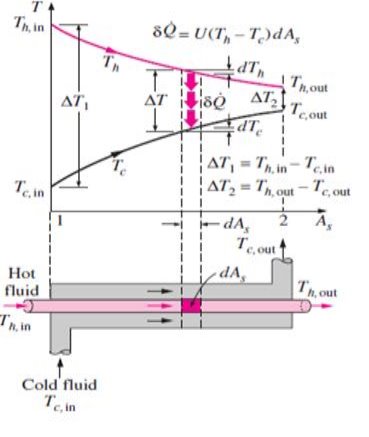
Fig: Variation of the fluid temperatures in a parallel-flow double-pipe heat exchanger.
δQ = mh Cph dTh ………………(1)
And
δQ = mc CpcdTc ………….(2)
That is, the rate of heat loss from the hot fluid at any section of a heat exchanger is equal to the rate of heat gain by the cold fluid in that section. The temperature change of the hot fluid is a negative quantity, and so a negative sign is added to
Eq. 1 to make the heat transfer rate Q a positive quantity.
Solving the equations above for dTh and dTc gives
DTh = δQ/(mhCph) ………(3)
And
DTc = δQ/(mcCpc) ………(4)
Taking their difference we get
d(Th – Tc) = δQ 1/(mhCph) + 1/(mcCpc) ……….(5)
The rate of heat transfer in the differential section of the heat exchanger can also be expressed as
δQ = U(Th – Tc) dAs
Substituting this equation into eq 5 and rearranging gives
Integrating from the inlet of the heat exchanger to its outlet, we obtain
From first law of thermodynamics requires that the rate of heat transfer from the hot fluid be equal to the rate of heat transfer to the cold one. That is,
Q = mcCpc(T(c, out)) – T(c, in))
And
Q = mh Cph(T(h, in)) – T(h, out))
Taking values of mCp from above equation and substituting in the integrating solution and soling it
Q =UAs Tlm
Where Tlm =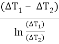 
Is the log mean temperature difference, which is the suitable form of the average temperature for use in the analysis of heat exchangers.
Q.9 a. State the ficks law of diffusion.
Answer:
Fick’s law of diffusion tells that the diffusion processes movement of molecules from higher concentration to lower concentration region. A diffusion process that obeys Fick’s laws is called normal diffusion or Fickian diffusion. A diffusion process that does not obey Fick’s laws is known as Anomalous diffusion or non-Fickian diffusion.
There are two laws are semiconductors i.e. Fick’s first law is used to derive Fick’s second law which is similar to the diffusion equation. According to Fick’s law of diffusion, “The molar flux due to diffusion is proportional to the concentration gradient”. The rate of change of concentration of the solution at a point in space is proportional to the second derivative of concentration with space.
Fick’s First Law
Movement of solute from higher concentration to lower concentration across a concentration gradient.
J = − D (dϕ / dx)
Where,
J: diffusion flux
D: diffusivity
Φ : concentration
x: position
b. Determine the view factor of the cylindrical surface with respect to the base, when L = 2r (Fig 2). Consider F12 = 0.16
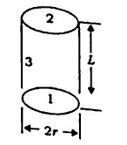
Answer:
For the surface-1:
F11 + F12 = 1
For the surface – 2:
F21 + F22 = 1
From the theorem of reciprocity,
A2F21 = A1F12
Given:
L = 2r
F12 = 0.16
Therefore F11 = 1 - 0.16
F11 = 0.84
C. Two infinite parallel plates are maintained at temperature t1 and t2 with t1>t2. To reduce the rate of
